|
Computer models This is how the downloadable WRCM works and also how the online Water Rocket Computer Model works - the image below is a simultaneous optimisation of water quantity and rocket weight for maximum downrange distance. 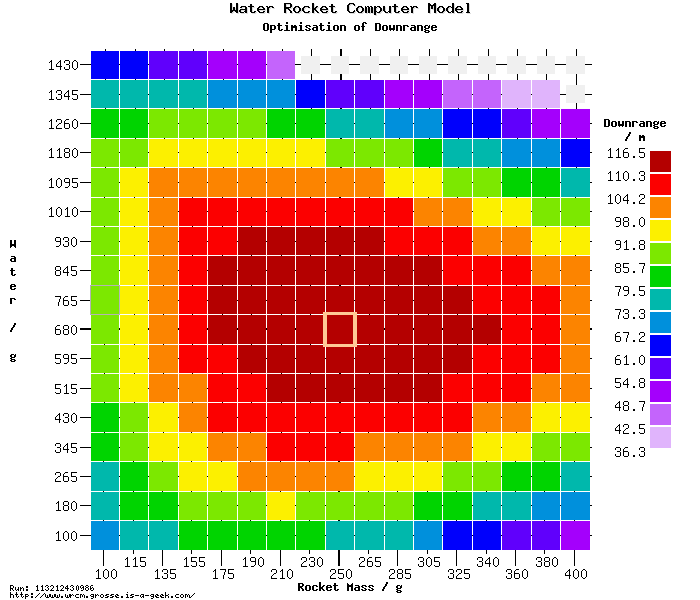 Why not use a straight forward mathematical equation and save all of this hassle? Some equations provide straight answers to simple questions such as how far will something have travelled after a particular amount of time has elapsed? The equation s = ut + Żat▓ gives the answer to this where: s = the distance; u = the initial velocity; t = the time interval; and, a = the acceleration. If you pick the right units, there will be no awkward constants - SI units are good enough for this. This is fine for rockets that only lose a little of their mass during the burn stage such as the solid fuel rockets used in toys and for these, there is Randy Culp's excellent page at http://www.execpc.com/~culp/rockets/rckt_eqn.html which gives details of solid fuel rocket flight equations - the larger solid fuel rockets, such as the two on the space shutte, are able to carry a greater proportion of fuel therefore suffering the same problems with calculations as the water rockets. With water rockets (as the big solid fuel boosters), around 90% of the weight disappears through the nozzle during the 'burn' (86% with the space shuttle boosters I am reliably told). The problem arises when you realise that in a water rocket, the acceleration is far from constant, changing all of the way through the 'burn' part of the flight and disappearing altogether after that. Figures for average mass or acceleration do not work in this case. Clearly, for water rockets, a model So, it looks like we are stuck with a computer model. Considerations Momentum and Drag A rocket is essentially a tank that is propelled upwards by the ejection of some mass. The acceleration of the ejected material produces an equal reaction in the opposite direction - pushing matter downwards, out of a rocket exhaust produces a reaction that tries to push the rocket upwards. During the course of the flight, the rockets momentum keeps it going until drag and gravity overcome it. In the case of a water rocket, the reaction mass is water and the force that is used to push is out comes from the pressure of the compressed air inside the rocket. The equation F = ma where F = the force; m = the mass; and, a = the acceleration may be used to calculate the acceleration of the rocket remembering that the forces balance each other out so that the mass of the reaction mass x the acceleration of the reaction mass = the mass of the rocket x the acceleration of the rocket or m pap = mrar However, this is not the complete picture as there are other forces on the rocket and it is simpler to group them together into upward and downward forces and perform a final sum. The other force considered that acts on the
rocket Flow of Reaction Mass One other thing to consider is how do we calculate the speed of the water (so that we can calculate its contribution to the momentum of the rocket). We know that the pressure inside the rocket forces out the water so we need to have an understanding of the way that gasses perform as well as how pressure is used to force a fluid through a pipe. As the water is forced out, the gas expands and the pressure drops - we need to know how much this happens to be able to predict the pressure for a given volume. Gas
Fluid I say fluid because it doesn't really matter if it is water or something else - try sea water. In a vertical system, the interface between the air and the water may be considered as a piston and any gas dissolving in the water ignored (Carbon Dioxide will dissolve and reduce the pressure in the rocket until it reaches equilibrium and if there is enough, it will effervesce as it depressurises upon passing through the nozzle making the viscosity and flow characteristics unpredictable). There are calculations that relate to the viscosity of a fluid but it has been found out by experiment that these effects, caused by turbulent flow, do not have a chance to establish themselves in the short distances operating in the nozzle of a water rocket - the pressure losses due to friction loss being negligable. The concentration of flow does, however, reduce the effective pressure drop along the nozzle and this does creep into the calculation as a constant dependent upon the internal geometry of the nozzle - a little like a drag factor but for the inside of the rocket. Knowing the velocity, we can calculate the mass of reaction mass and its contribution to the momentum of the rocket system. By calculating through a set of equations, we are able to work out where in the sky the rocket will be, how fast it is travelling and various other values. Limitations For a water rocket designed in the middle of the effective range, with all calibrations perfected, the flight should be predicted correctly. However, errors start to creep into things throughout the process when there is too great a deviation from this calibrated norm. For the distances and speeds travelled, Newtonian mechanics is good enough - we are hardly likely to encounter a significant manifestation of the twins paradox with a water rocket (it actually works out to be around 4fs that is 0.000 000 000 000 004 seconds accumulated time dilation over the trip; for anyone looking upwards at its maximum speed, gives a red shift of around 0.1ppm; and, applying the Lorentz transformation makes its length contract by around 1fm for the stationary observer although the effects of external air pressure on the rocket body during flight will make it shrink more than this). For it to work, Water, travelling at velocity 1 is accelerated to velocity 2. If the ratio of the diameters of the body of the rocket to the nozzle is sufficiently high, then velocity 1 may be ignored. However, the model takes this into account therefore smaller constriction ratios may be used. This means that the thrust will vary according to the dry weight of the rocket because the lighter the rocket, the more the acceleration, the greater the pressure at the nozzle, the greater the thrust, the more the acceleration . . . Just to make this more interesting, the air on
the outside is also: compressible; changes its
pressure and temperature (and viscosity)
at different heights; and; has movement in it.
Hmmph. The equations themselves make certain assumptions: assuming that the equations describe accurately the effects of pressure on the fluid; the fact that the rocket will change its size when the pressure inside it alters during flight along with the pressure on the front of the rocket that causes drag; the fact that the ratio of specific heat capacity at constant pressure and volume is not a constant at all the effect of the vapour pressure of the reaction mass on the dew point of the gas and its effect on the specific heat capacities; the fact that the coefficient of drag changes slightly over the range of speeds of a water rocket; and so on. Many of these effects are small and can be
ignored - the errors encountered when flying the
real rocket will by far exceed any of these.
Working out the pressure that you deliver to the
rocket is a problem if you have not got a
pressure gauge. For discussion on the topic of improving flights - assembly of more advanced rockets, glues, lauchers (a swing arm launcher can't possibly be as dangerous as it sounds) delivery of compressed gas, release mechanisms and so on, join in on e-mail discussion on http://www.h2orocket.com/email.html or http://www.osa.com.au/~cjh/rockets/list.html In Summary We can perform complex calculations that are unfeasible to express as a single equation by following a series of logical steps which go as follows . . .
Hopefully, this process allows the real water rocket's flight to be predicted reasonably accurately. Put in a parachute and it looks a little more complicated than this :-) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
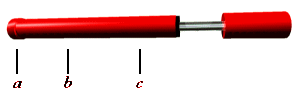 You
know that the end of the bicycle pump (in the
diagram) is at point a and
that you started the pump stroke at point c
and that the one-way valve started letting air
through at point b
and from this, you can work out the pressure in
the rocket if you take into account the fact that
you don't have a particularly good idea of where
the piston really is and you don't know what
pressure is needed to open the one-way valve. Did
you really need to know the height of the flight
to the nearest foot when, during the real flight,
it shot off at 45░ and landed in a neighbour's
garden? or that you forgot to make sure that the
water inside was still and a vortex appeared
allowing all of the gas to escape with 20%
You
know that the end of the bicycle pump (in the
diagram) is at point a and
that you started the pump stroke at point c
and that the one-way valve started letting air
through at point b
and from this, you can work out the pressure in
the rocket if you take into account the fact that
you don't have a particularly good idea of where
the piston really is and you don't know what
pressure is needed to open the one-way valve. Did
you really need to know the height of the flight
to the nearest foot when, during the real flight,
it shot off at 45░ and landed in a neighbour's
garden? or that you forgot to make sure that the
water inside was still and a vortex appeared
allowing all of the gas to escape with 20%